Skip to main content
SpringerLink
Log in

The incompressible limit method and Rayleigh waves in incompressible layered nonlocal orthotropic elastic media

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

A Correction to this article was published on 12 November 2022

This article has been updated

Abstract

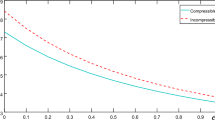
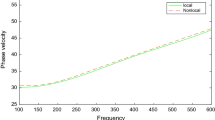
In this paper, we introduce the incompressible limit method to nonlocal orthotropic elastic solids under plane strain. Then, applying it we investigate Rayleigh waves propagating in a nonlocal orthotropic elastic half-space coated with a nonlocal orthotropic elastic layer in which the layer and the half-space may be compressible or incompressible, and they are in welded contact with each other. Our main aim is to derive explicit secular equations of Rayleigh waves. These secular equations are derived using the nonlocal stress boundary conditions, not the local stress ones as previously used. First, the secular equation for the compressible case (both the half-space and the layer are compressible) is derived by using the effective boundary condition technique. Then, the secular equations for the incompressible cases (at least one of the half-space and the layer is incompressible) are obtained by taking the incompressible limit of the obtained compressible secular equation. The simple and immediate derivation of the incompressible secular equations proves the convenience and powerfulness of the incompressible limit technique. Some numerical examples are carried out to show the strong effect of the incompressibility and the nonlocality on the Rayleigh wave velocity. Remarkably, while the nonlocality of half-spaces makes the Rayleigh wave velocity decreasing, the nonlocality of layers can increase the Rayleigh wave velocity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Change history

References

  1. Iijima, S.: Helical microtubules of graphitic carbon. Nature 354, 56–58 (1991)

    Article  Google Scholar 

  2. Yan, J.W., Liew, K.M., He, L.H.: A higher-order gradient theory for modeling of the vibration behavior of single-wall carbon nanocones. Appl. Math. Model. 38, 2946–2960 (2014)

    Article  MathSciNet  Google Scholar 

  3. Hoe, Y.S., Jaafar, C.L., Yong, N.T.: Molecular dynamics modeling and simulations of carbon nanotube-based gears. Sains Malays. 41, 901–906 (2012)

    Google Scholar 

  4. Chowdhury, S.C., Haque, B.Z., Gillespie, J.W., Hartman, D.R.: Molecular simulations of pristine and defective carbon nanotubes under monotonic and combined loading. Comput. Mater. Sci. 65, 133–143 (2012)

    Article  Google Scholar 

  5. Eringen, A.C., Edelen, D.G.B.: On nonlocal elasticity. Int. J. Eng. Sci. 10, 233–248 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  6. Eringen, A.C.: Linear theory of nonlocal elasticity and dispersion of plane waves. Int. J. Eng. Sci. 10, 425–435 (1972)

    Article  MATH  Google Scholar 

  7. Eringen, A.C.: On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J. Appl. Phys. 54, 4703–4710 (1983)

    Article  Google Scholar 

  8. Eringen, A.C.: Theory of nonlocal elasticity and some applications. Res. Mech. 21, 313–342 (1987)

    Google Scholar 

  9. Eringen, A.C.: Nonlocal Continuum Field Theories. Springer, New York (2002)

    MATH  Google Scholar 

  10. Eringen, A.C.: Nonlocal polar elastic continua. Int. J. Eng. Sci. 10, 1–16 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  11. Eringen, A.C.: Plane waves in nonlocal micropolar elasticity. Int. J. Eng. Sci. 22, 1113–1121 (1984)

    Article  MATH  Google Scholar 

  12. Zhang, Q., Sun, Y., Yang, J.: Propagation and reflection of plane waves in biological tissue based on nonlocal TPL thermoelasticity. Int. Commun. Heat Mass Transf. 128, 105587 (2021)

    Article  Google Scholar 

  13. Bachher, M., Sarkar, N.: Nonlocal theory of thermoelastic materials with voids and fractional derivative heat transfer. Waves Random Complex Media 29, 595–613 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  14. McCay, B.M., Narsimhan, M.L.N.: Theory of nonlocal electromagnetic fluids. Arch. Mech. 33, 365–384 (1981)

    MathSciNet  MATH  Google Scholar 

  15. Eringen, A.C.: Memory-dependent nonlocal electromagnetic elastic solids and superconductivity. J. Math. Phys. 32, 787–796 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  16. Eringen, A.C.: On nonlocal fluid mechanics. Int. J. Eng. Sci. 10, 561–575 (1972)

    Article  MATH  Google Scholar 

  17. Eringen, A.C.: Nonlocal continuum theory of liquid crystals. Mol. Cryst. Liq. Cryst. 75, 321–343 (1981)

    Article  Google Scholar 

  18. Singh, D., Kaur, G., Tomar, S.K.: Waves in nonlocal elastic solid with voids. J. Elast. 128, 85–114 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  19. Chakraborty, A.: Prediction of negative dispersion by a nonlocal poroelastic theory. J. Acoust. Soc. Am. 123, 56–67 (2008)

    Article  Google Scholar 

  20. Tong, L.H., Yu, Y., Hu, W., Shi, Y., Xu, C.: On wave propagation characteristics in fluid saturated porous materials by a nonlocal Biot theory. J. Sound Vib. 379, 106–118 (2016)

    Article  Google Scholar 

  21. Peddieson, J., Buchanan, G.R., McNitt, R.P.: Application of nonlocal continuum models to nanotechnology. Int. J. Eng. Sci. 41, 305–312 (2003)

    Article  Google Scholar 

  22. Reddy, J.N., Pang, S.D.: Nonlocal continuum theories of beams for the analysis of carbon nanotubes. J. Appl. Phys. 103, 023511 (2008)

    Article  Google Scholar 

  23. Ghannadpour, S.A.M., Mohammadi, B., Fazilati, J.: Bending, buckling and vibration problems of nonlocal Euler beams using Ritz method. Compos. Struct. 96, 584–589 (2013)

    Article  Google Scholar 

  24. Yan, J.W., Tong, L.H., Li, C., Zhu, Y., Wang, Z.W.: Exact solutions of bending deflections for nano-beams and nano-plates based on nonlocal elasticity theory. Compos. Struct. 125, 304–313 (2015)

    Article  Google Scholar 

  25. Aghababaei, R., Reddy, J.N.: Nonlocal third-order shear deformation plate theory with application to bending and vibration of plates. J. Sound Vib. 326, 277–289 (2009)

    Article  Google Scholar 

  26. Reddy, J.N.: Nonlocal nonlinear formulations for bending of classical and shear deformation theories of beams and plates. Int. J. Eng. Sci. 48, 1507–1518 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  27. Phadikar, J.K., Pradhan, S.C.: Variational formulation and finite element analysis for nonlocal elastic nanobeams and nanoplates. Comput. Mater. Sci. 49, 492–499 (2010)

    Article  Google Scholar 

  28. Wang, Q., Varadan, V.K.: Application of nonlocal elastic shell theory in wave propagation analysis of carbon nanotubes. Smart Mater. Struct. 16, 178–190 (2007)

    Article  Google Scholar 

  29. Hu, Y.G., Liew, K.M., Wang, Q., He, X.Q., Yakobson, B.I.: Nonlocal shell model for elastic wave propagation in single- and double-walled carbon nanotubes. J. Mech. Phys. Solids 56, 3475–3485 (2008)

    Article  MATH  Google Scholar 

  30. Duan, W.H., Wang, C.M.: Exact solutions for axisymmetric bending of micro/nanoscale circular plates based on nonlocal plate theory. Nanotechnology 18, 385704 (2007)

    Article  Google Scholar 

  31. Huang, L.Y., Han, Q., Liang, Y.J.: Calibration of nonlocal scale effect parameter for bending single-layered graphene sheet under molecular dynamics. Nano Brief Rep. Rev. 7, 1250033 (2012)

    Google Scholar 

  32. Wang, Q., Varadan, V.K.: Vibration of carbon nanotubes studied using nonlocal continuum mechanics. Smart Mater. Struct. 15, 659–666 (2006)

    Article  Google Scholar 

  33. Wang, C.M., Zhang, Y.Y., He, X.Q.: Vibration of nonlocal Timoshenko beams. Nanotechnology 18, 105401 (2007)

    Article  Google Scholar 

  34. Pradhan, S.C., Phadikar, J.K.: Nonlocal elasticity theory for vibration of nanoplates. J. Sound Vib. 325, 206–223 (2009)

    Article  Google Scholar 

  35. Aksencer, T., Aydogdu, M.: Levy type solution method for vibration and buckling of nanoplates using nonlocal elasticity theory. Physica E 43, 954–959 (2011)

    Article  Google Scholar 

  36. Pradhan, S.C.: Buckling of single layer graphene sheet based on nonlocal elasticity and higher order shear deformation theory. Phys. Lett. A 373, 4182–4188 (2009)

    Article  MATH  Google Scholar 

  37. Ansari, R., Rouhi, H.: Explicit analytical expressions for the critical buckling stresses in a monolayer graphene sheet based on nonlocal elasticity. Solid State Commun. 152, 56–59 (2012)

    Article  Google Scholar 

  38. Verma, K.L.: Nonlocal wave surfaces in elastic solids. Ann. Faculty Eng. Hunedoara Int. J. Eng. Tome 19 (Fascicule 2, May), 41–46 (2021)

  39. Singh, B.: Propagation of waves in an incompressible rotating transversely isotropic nonlocal solid. Vietnam J. Mech. 43, 237–252 (2021)

    Google Scholar 

  40. Acharya, D.P., Mondal, A.: Propagation of Rayleigh surface waves with small wavelengths in nonlocal visco-elastic solids. Sadhana 27, 605–612 (2002)

    Article  MATH  Google Scholar 

  41. Das, N., Sarkar, N., Lahiri, A.: Reflection of plane waves from the stress-free isothermal and insulated boundaries of a nonlocal thermoelastic solid. Appl. Math. Model. 73, 526–544 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  42. Pramanik, A.S., Biswas, S.: Surface waves in nonlocal thermoelastic medium with state space approach. J. Therm. Stress. 43, 667–686 (2020)

    Article  Google Scholar 

  43. Yan, D.J., Chen, A.L., Wang, Y.S.H., Zhang, C., Golub, M.: Propagation of guided elastic waves in nanoscale layered periodic piezoelectric composites. Eur. J. Mech. A/Solids 66, 158–167 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  44. Yan, D.J., Chen, A.L., Wang, Y.S.H., Zhang, C., Golub, M.: In-plane elastic wave propagation in nanoscale periodic layered piezoelectric structures. Int. J. Mech. Sci. 142–143, 276–288 (2018)

    Article  Google Scholar 

  45. Tung, D.X.: Dispersion equation of Rayleigh waves in transversely isotropic nonlocal piezoelastic solids half-spaces. Vietnam J. Mech. 41, 363–371 (2019)

    Article  Google Scholar 

  46. Liu, C., Yu, J., Wang, X., Zhan, B., Zhang, X., Zhou, H.: Reflection and transmission of elastic waves through nonlocal piezoelectric plates sandwiched in two solid half-spaces. Thin-Walled Struct. 168, 108306 (2021)

    Article  Google Scholar 

  47. Khurana, A., Tomar, S.K.: Reflection of plane longitudinal waves from the stress-free boundary of a nonlocal, micropolar solid half-space. J. Mech. Mater. Struct. 8, 95–107 (2013)

    Article  Google Scholar 

  48. Khurana, A., Tomar, S.K.: Rayleigh-type waves in nonlocal micropolar solid half-space. Ultrasonics 73, 162–168 (2017)

    Article  Google Scholar 

  49. Khurana, A., Tomar, S.K.: Waves at interface of dissimilar nonlocal micropolar elastic half-spaces. Mech. Adv. Mater. Struct. 26, 825–833 (2019)

    Article  Google Scholar 

  50. Kalka, K.K., Sheoran, D., Deswal, S.: Reflection of plane waves in a nonlocal micropolar thermoelastic medium under the effect of rotation. Acta Mech. 231, 2849–2866 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  51. Kumar, S., Tomar, S.K.: Plane waves in nonlocal micropolar thermoelastic material with voids. J. Therm. Stress. 43, 1355–1378 (2020)

    Article  Google Scholar 

  52. Deswal, S., Sheoran, D., Thakran, S., Kalkal, K.K.: Reflection of plane waves in a nonlocal microstretch thermoelastic medium with temperature dependent properties under three-phase-lag model. Mech. Adv. Mater. Struct. (2020). https://doi.org/10.1080/15376494.2020.1837307

    Article  MATH  Google Scholar 

  53. Tung, D.X.: The reflection and transmission of a quasi-longitudinal displacement wave at an imperfect interface between two nonlocal orthotropic micropolar half-spaces. Arch. Appl. Mech. 91, 4313–4328 (2021)

    Article  Google Scholar 

  54. Tung, D.X.: Wave propagation in nonlocal orthotropic micropolar elastic solids. Arch. Mech. 73, 237–251 (2021)

    MathSciNet  MATH  Google Scholar 

  55. Tong, L.H., Lai, S.K., Zeng, L.L., Xu, C.J., Yang, J.: Nonlocal scale effect on Rayleigh wave propagation in porous fluid-saturated materials. Int. J. Mech. Sci. 148, 459–466 (2018)

    Article  Google Scholar 

  56. Tung, D.X.: The reflection and transmission of waves at an imperfect interface between two nonlocal transversely isotropic liquid-saturated porous half-spaces. Waves Random Complex Media (2021). https://doi.org/10.1080/17455030.2021.1954265

    Article  Google Scholar 

  57. Tung, D.X.: Surface waves in nonlocal transversely isotropic liquid-saturated porous solid. Arch. Appl. Mech. 91, 2881–2892 (2021)

    Article  Google Scholar 

  58. Kaur, G., Singh, D., Tomar, S.K.: Love waves in a nonlocal elastic media with voids. J. Vib. Control (2019). https://doi.org/10.1080/17455030.2021.1954265

    Article  MathSciNet  Google Scholar 

  59. Sarkar, N., Tomar, S.K.: Plane waves in nonlocal thermoelastic solid with voids. J. Therm. Stress. 42, 580–606 (2019)

    Article  Google Scholar 

  60. Kaur, G., Singha, D., Tomar, S.K.: Lamb waves in nonlocal elastic with voids. J. Mech. Mater. Struct. 16, 389–405 (2020)

    Article  MathSciNet  Google Scholar 

  61. Kaur, G., Singh, D., Tomar, S.K.: Rayleigh-type wave in a nonlocal elastic solid with voids. Eur. J. Mech./A Solids 71, 134–150 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  62. Abd-Alla, A.M., Abo-Dahab, S.M., Ahmed, S.M., Rashid, M.M.: Effect of magnetic field and voids on Rayleigh waves in a nonlocal thermoelastic half-space. J. Strain Anal. Eng. Des. (2021). https://doi.org/10.1177/03093247211001243

    Article  Google Scholar 

  63. Gill, K.S., Sawhney, S.: Rayleigh waves with impedance boundary conditions in a nonlocal micropolar thermoelastic material. J. Phys: Conf. Ser. 1531, 012048 (2020)

    Google Scholar 

  64. Kaur, B., Singh, B.: Rayleigh-type surface wave in nonlocal isotropic diffusive materials. Acta Mech. 232, 3407–3416 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  65. Biswas, S.: Rayleigh waves in a nonlocal thermoelastic layer lying over a nonlocal thermoelastic half-space. Acta Mech. 231, 4129–4144 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  66. Ogden, R.W.: Elastic deformations of rubberlike solids. In: Hopkins, H.G., Sewell, M.J. (eds.) Mechanics of Solids. The Rodney Hill 60th Anniversary Volume, pp. 499–537. Pergamon Press, Oxford (1982)

    Google Scholar 

  67. Amabil, M., Breslavsky, I.D., Reddy, J.D.: Nonlinear higher-order shell theory for incompressible biological hyperelastic materials. Comput. Methods Appl. Mech. Eng. 346, 841–861 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  68. Vinh, P.C.: Explicit secular equations of Rayleigh waves in elastic media under the influence of gravity and initial stress. Appl. Math. Comp 215, 395–404 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  69. Vinh, P.C., Seriani, G.: Explicit secular equations of Stoneley waves in a non-homogeneous orthotropic elastic medium under the influence of gravity. Appl. Math. Comp 215, 3515–3525 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  70. Vinh, P.C., Anh, V.T.N., Linh, N.T.K.: Exact secular equations of Rayleigh waves in an orthotropic elastic half-space overlaid an orthotropic elastic layer. Int. J. Solds Struct. 83, 65–72 (2016)

    Article  Google Scholar 

  71. Vinh, P.C., Ogden, R.W.: Formulas for the Rayleigh wave speed in orthotropic elastic solids. Arch. Mech. 56, 247–265 (2004)

    MathSciNet  MATH  Google Scholar 

  72. Ting, T.C.T.: Anisotropic Elasticity Theory and Applications. Oxford University Press, New York (1996)

    Book  MATH  Google Scholar 

  73. Ogden, R.W., Vinh, P.C.: On Rayleigh waves in incompressible orthotropic elastic solids. J. Acoust. Soc. Am. 115, 530–533 (2004)

    Article  Google Scholar 

Download references

Acknowledgements

Vu Thi Ngoc Anh was funded by Vingroup JSC and supported by the Postdoctoral Scholarship Programme of Vingroup Innovation Foundation (VINIF), Institute of Big Data, Code VINIF.2021.STS.02.

Author information

Authors and Affiliations

  1. Faculty of Mathematics, Mechanics and Informatics, University of Science, Vietnam National University, Hanoi, 334, Nguyen Trai Str., Thanh Xuan, Hanoi, Vietnam

    V. T. N. Anh & P. C. Vinh

Authors
  1. V. T. N. Anh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. C. Vinh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to P. C. Vinh.

Ethics declarations

Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The original online version of this article was revised: the uncorrected errors have been corrected" plus the same explanatory text of the problem as in the erratum/correction article.

Appendices

Appendix A: The coefficients of the secular equation (48)

$$\begin{aligned} \bar{A}_0= & {} 2\bar{\beta }_1^*\bar{\beta }_2^*\bar{\gamma }_1^*\bar{\gamma }_2^*[\alpha ^*] -[\bar{\alpha }^*;\bar{\beta }^*\bar{\gamma }^*]^+[\gamma ^*;\beta ^*]-\bar{\gamma }_1^*\bar{\gamma }_2^*[\bar{\alpha }^*;\bar{\beta }^*]^+[\beta ^*]-\bar{\beta }_1^*\bar{\beta }_2^*[\bar{\gamma }^*]^+[\alpha ^*;\gamma ^*],\nonumber \\ \bar{B}_0= & {} [\bar{\beta }^{*2};\bar{\gamma }^{*2}]^+[\alpha ^*]-[\bar{\alpha }^*\bar{\beta }^*;\bar{\gamma }^*]^+[\gamma ^*;\beta ^*]-[\bar{\alpha }^*\bar{\beta }^*;\bar{\gamma }^{*2}]^+[\beta ^*]-[\bar{\beta }^{*2};\bar{\gamma }^*]^+[\alpha ^*;\gamma ^*],\nonumber \\ \bar{C}_0= & {} \bar{\beta }_2^*\bar{\gamma }_1^*[\bar{\gamma }^*][\alpha ^*;\beta ^*]-\bar{\beta }_1^*\bar{\gamma }_2^* [\bar{\alpha }^*;\bar{\beta }^*][\gamma ^*],\, \bar{D}_0=-\bar{\beta }_1^*\bar{\gamma }_2^*[\bar{\gamma }^*][\alpha ^*;\beta ^*]+\bar{\beta }_2^*\bar{\gamma }_1^* [\bar{\alpha }^*;\bar{\beta }^*][\gamma ^*],\nonumber \\ \bar{E}_0= & {} -\bar{A}_0+[\bar{\gamma }^*][\bar{\alpha }^*;\bar{\beta }^*][\gamma ^*;\beta ^*], \end{aligned}$$
(68)

where:

$$\begin{aligned} \bar{\alpha }_j^*&=\,\dfrac{(\bar{e}_3+1)\bar{s}_j}{1-\bar{e}r_v^2x-(\bar{e}_2-\bar{e}r_v^2x)\bar{s}_j^2},\,\bar{\beta }_j^*=\dfrac{r_\mu (\bar{s}_j-\bar{\alpha }^*_j)}{1+\bar{e}(1-\bar{s}_j^2)},\,\bar{\gamma }_j^*=r_\mu \dfrac{\bar{e}_3+\bar{e}_2\bar{s}_j\bar{\alpha }^*_j}{1+\bar{e}(1-\bar{s}_j^2)},\nonumber \\ [\alpha ^*]&=\,\theta ^*\Big [x-e_1+ex-(1-ex)\sqrt{P} \Big ],\nonumber \\ [\beta ^*]&=\,-{(1+e)\big [x-e_1+ex+(e_3+xe)\sqrt{P} \big ]- e \big [(e_1-ex-x)(S+\sqrt{P})+(e_3+xe)P \big ] } ,\nonumber \\ [\gamma ^*]&=\,\Big \{(1+e)e_2(1-ex)+e\big [e_3(e_3+1)-e_2(e_1-ex-x) \big ] \Big \} \sqrt{P}\sqrt{S+2\sqrt{P}},\nonumber \\ [\alpha ^*;\beta ^*]&=\,(e_1-ex-x)\Big [1+\dfrac{e}{ e_2-ex}(e_2+e_3+x) \Big ]\sqrt{S+2\sqrt{P}},\nonumber \\ [\alpha ^*;\gamma ^*]&=\, (1+e)\Big [\dfrac{e_1-ex-x}{e_2-ex}(ee_3x+e_2)-e_3(1-ex)\sqrt{P} \Big ]\nonumber \\&+e\sqrt{P}\Big [\dfrac{e_1-ex-x}{e_2-ex}(e_3xe+e_2)+e_3(1-ex)(S+\sqrt{P}) \Big ],\nonumber \\ [\gamma ^*;\beta ^*]&=\, \big [e_3^2-e_2(e_1-ex-x)+ee_3x\big ]\sqrt{P}+ x(e_1-ex-x) \dfrac{e_2(1+e)+ee_3}{e_2-ex} \end{aligned}$$
(69)

in which:

$$\begin{aligned} \begin{aligned} S=&\,\dfrac{[ e_1-x(1+e)](e_2-ex)+[1-x(1+e)](1-ex)-(e_3+1)^2}{(1-ex)(e_2-ex)},\\ P=&\,\dfrac{[e_1-x(1+e)][1-x(1+e)]}{(1-ex)(e_2-ex)}, \end{aligned} \end{aligned}$$
(70)

and \(\bar{s}_j\,(j=1,2)\) are determined by (18) with \(\bar{S}\) and \(\bar{P}\) being calculated by:

$$\begin{aligned} \begin{aligned} \bar{S}=&\,\dfrac{[ \bar{e}_1-r_v^2x(1+\bar{e})](\bar{e}_2-\bar{e}r_v^2x)+[1-r_v^2x(1+\bar{e})](1-\bar{e}r_v^2x)-(\bar{e}_3+1)^2}{(1-\bar{e}r_v^2x)(\bar{e}_2-\bar{e}r_v^2x)},\\ \bar{P}=&\,\dfrac{[\bar{e}_1-r_v^2x(1+\bar{e})][1-r_v^2x(1+\bar{e})]}{(1-\bar{e}r_v^2x)(\bar{e}_2-\bar{e}r_v^2x)}, \end{aligned} \end{aligned}$$
(71)

Appendix B: The coefficients of the secular equation (62)

$$\begin{aligned} \hat{A}_0&=2\bar{\beta }_1'\bar{\beta }_2'\bar{\gamma }_1'\bar{\gamma }_2'[\alpha ^*] +[\bar{\alpha }';\bar{\beta }'\bar{\gamma }']^+[\gamma ^*;\beta ^*]+\bar{\gamma }_1'\bar{\gamma }_2'[\bar{\beta }']^+[\beta ^*]-\bar{\beta }_1'\bar{\beta }_2'[\bar{\alpha }';\bar{\gamma }']^+[\alpha ^*;\gamma ^*],\nonumber \\ \hat{B}_0&=[\bar{\beta }'^2;\bar{\gamma }'^2]^+[\alpha ^*]+[\bar{\alpha }'\bar{\gamma }';\bar{\beta }']^+[\gamma ^*;\beta ^*]+[\bar{\beta }';\bar{\gamma }'^2]^+[\beta ^*]-[\bar{\alpha }'\bar{\gamma }';\bar{\beta }'^2]^+[\alpha ^*;\gamma ^*], \nonumber \\ \hat{C}_0&=-\bar{\beta }_2'\bar{\gamma }_1'[\bar{\alpha }';\bar{\gamma }'][\alpha ^*;\beta ^*]-\bar{\beta }_1'\bar{\gamma }_2' [\bar{\beta }'][\gamma ^*],\, \hat{D}_0=\bar{\beta }_1'\bar{\gamma }_2'[\bar{\alpha }';\bar{\gamma }'][\alpha ^*;\beta ^*]+\bar{\beta }_2'\bar{\gamma }_1' [\bar{\beta }'][\gamma ^*],\nonumber \\ \hat{E}_0&=-\hat{A}_0-[\bar{\alpha }';\bar{\gamma }'][\bar{\beta }'][\gamma ^*;\beta ^*], \end{aligned}$$
(72)

here \(\bar{\alpha }'_j=\bar{s}_j\), \(\bar{\beta }_j',\,\bar{\gamma }_j'\) are determined by (55), and: \([\alpha ^*],\,[\beta ^*],\,[\gamma ^*],\,[\alpha ^*,\beta ^*],\,[\alpha ^*;\gamma ^*],\,[\gamma ^*;\beta ^*]\) are given by (69).

Appendix C: The coefficients of the secular equation (64)

$$\begin{aligned} \hat{A}_0&=2\bar{\beta }_1'\bar{\beta }_2'\bar{\gamma }_1'\bar{\gamma }_2'[\alpha ']+[\bar{\alpha }';\bar{\beta }'\bar{\gamma }']^+[\gamma ';\beta ']+\bar{\gamma }_1'\bar{\gamma }_2'[\bar{\beta }']^+[\beta ']-\bar{\beta }_1'\bar{\beta }_2'[\bar{\alpha }';\bar{\gamma }']^+[\alpha ';\gamma '],\nonumber \\ \hat{B}_0&=[\bar{\beta }'^2;\bar{\gamma }'^2]^+[\alpha ']+[\bar{\alpha }'\bar{\gamma }';\bar{\beta }']^+[\gamma ';\beta ']+[\bar{\beta }';\bar{\gamma }'^2]^+[\beta ']-[\bar{\alpha }'\bar{\gamma }';\bar{\beta }'^2]^+[\alpha ';\gamma '],\nonumber \\ \hat{C}_0&=-\bar{\beta }_2'\bar{\gamma }_1'[\bar{\alpha }';\bar{\gamma }'][\alpha ';\beta ']-\bar{\beta }_1'\bar{\gamma }_2' [\bar{\beta }'][\gamma '],\,\,\hat{D}_0=\bar{\beta }_1'\bar{\gamma }_2'[\bar{\alpha }';\bar{\gamma }'][\alpha ';\beta ']+\bar{\beta }_2'\bar{\gamma }_1' [\bar{\beta }'][\gamma '],\nonumber \\ \hat{E}_0&=-\hat{A}_0-[\bar{\alpha }';\bar{\gamma }'][\bar{\beta }'][\gamma ';\beta '], \end{aligned}$$
(73)

where \(\bar{\alpha }'_j=\bar{s}_j\), \(\bar{\beta }_j',\,\bar{\gamma }_j'\) are determined by (55), and \([\alpha '],\,[\beta '],\,[\gamma '],\,[\alpha ',\beta '],\,[\alpha ';\gamma '],\,[\gamma ';\beta ']\) are given by (61).

Appendix D: The coefficients of the secular equation (66)

$$\begin{aligned} \hat{A}_0&=2\bar{\beta }_1^*\bar{\beta }_2^*\bar{\gamma }_1^*\bar{\gamma }_2^*[\alpha '] -[\bar{\alpha }^*;\bar{\beta }^*\bar{\gamma }^*]^+[\gamma ';\beta ']-\bar{\gamma }_1^*\bar{\gamma }_2^*[\bar{\alpha }^*;\bar{\beta }^*]^+[\beta ']-\bar{\beta }_1^*\bar{\beta }_2^*[\bar{\gamma }^*]^+[\alpha ';\gamma '], \nonumber \\ \hat{B}_0&=[\bar{\beta }^{*2};\bar{\gamma }^{*2}]^+[\alpha ']-[\bar{\alpha }^*\bar{\beta }^*;\bar{\gamma }^*]^+[\gamma ';\beta ']-[\bar{\alpha }^*\bar{\beta }^*;\bar{\gamma }^{*2}]^+[\beta ']-[\bar{\beta }^{*2};\bar{\gamma }^*]^+[\alpha ';\gamma '], \nonumber \\ \hat{C}_0&=\bar{\beta }_2^*\bar{\gamma }_1^*[\bar{\gamma }^*][\alpha ';\beta ']-\bar{\beta }_1^*\bar{\gamma }_2^* [\bar{\alpha }^*;\bar{\beta }^*][\gamma '],\, \hat{D}_0=-\bar{\beta }_1^*\bar{\gamma }_2^*[\bar{\gamma }^*][\alpha ';\beta ']+\bar{\beta }_2^*\bar{\gamma }_1^* [\bar{\alpha }^*;\bar{\beta }^*][\gamma '], \nonumber \\ \hat{E}_0&=-\hat{A}_0+[\bar{\gamma }^*][\bar{\alpha }^*;\bar{\beta }^*][\gamma ';\beta '], \end{aligned}$$
(74)

where \(\bar{\alpha }_j^*,\,\bar{\beta }_j^*,\,\bar{\gamma }_j^*\) are determined by (69), and: \([\alpha '],\,[\beta '],\,[\gamma '],\,[\alpha ',\beta '],\,[\alpha ';\gamma '],\,[\gamma ';\beta ']\) are given by (61).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Anh, V.T.N., Vinh, P.C. The incompressible limit method and Rayleigh waves in incompressible layered nonlocal orthotropic elastic media. Acta Mech 234, 403–421 (2023). https://doi.org/10.1007/s00707-022-03319-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-022-03319-y

Search

Navigation